反三角函数是数学学习中一个很重要的知识点,下面整理了相关知识点和公式,希望能帮助到大家。

设函数y=f(x)的定义域是A,值域是C.我们从式子y=f(x)中解出x得到式子x=φ(y).如果对于y在C中的任何一个值,通过式子x=φ(y),x在A中都有唯一的值和它对应,那么式子x=φ(y)叫函数y=f(x)的反函数,记作x=f-1(y),习惯表示为y=f-1(x)。注意:函数y=f(x)的定义域和值域,分别是反函数y=f-1(x)的值域和定义域。
例如:f(x)的定义域是[-1,+∞],值域是[0,+∞),它的反函数定义域为[0,+∞),值域是[-1,+∞)。
余角关系
arcsin(x)+arccos(x)=π/2
arctan(x)+arccot(x)=π/2
arcsec(x)+arccsc(x)=π/2
负数关系
arcsin(-x)=-arcsin(x)
arccos(-x)=π-arccos(x)
arctan(-x)=-arctan(x)
arccot(-x)=π-arccot(x)
arcsec(-x)=π-arcsec(x)
arccsc(-x)=-arccsc(x)
cos(arcsinx)=√(1-x^2)
arcsin(-x)=-arcsinx
arccos(-x)=π-arccosx
arctan(-x)=-arctanx
arccot(-x)=π-arccotx
arcsinx+arccosx=π/2=arctanx+arccotx
sin(arcsinx)=cos(arccosx)=tan(arctanx)=cot(arccotx)=x
当x∈[-π/2,π/2]有arcsin(sinx)=x
x∈[0,π],arccos(cosx)=x
x∈(-π/2,π/2),arctan(tanx)=x
x∈(0,π),arccot(cotx)=x
x>0,arctanx=π/2-arctan1/x,arccotx类似
若(arctanx+arctany)∈(-π/2,π/2),则arctanx+arctany=arctan((x+y)/(1-xy))

反三角函数是一种基本初等函数。它是反正弦arcsinx,反余弦arccosx,反正切arctanx,反余切arccotx,反正割arcsec...

反三角函数是一种基本初等函数。小编给大家整理了反三角函数公式及图像,希望对同学们学习有帮助。

反三角函数是一种基本初等函数。接下来小编给大家总结了有关反正弦三角函数的公式,一起看一下具体内容,供参考。

反三角函数是一种基本初等函数,是数学学习中一个很重要的知识点,下面整理了相关知识点和公式,希望能帮助到大家。

反三角函数定义域是反三角函数一个重要的知识点,下面总结了反三角函数的定义域,供大家参考。

反三角函数是一种基本初等函数。这篇文章给大家分享反三角函数的计算公式,一起看一下具体内容。
反三角函数是数学学习中一个很重要的知识点,下面整理了相关知识点和公式,希望能帮助到大家。
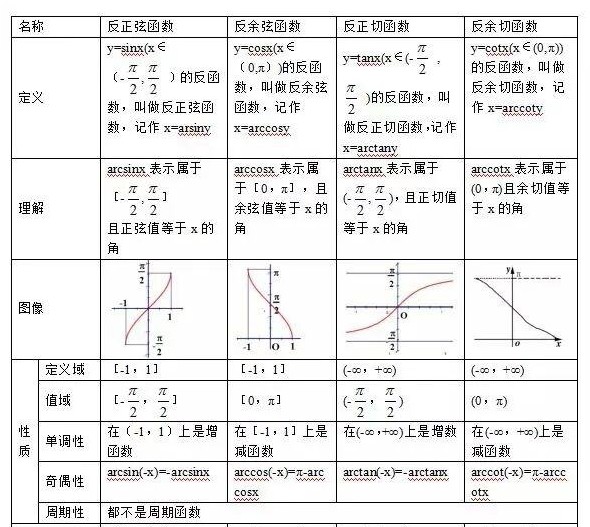
反三角函数是反正弦arcsinx,反余弦arccosx,反正切arctanx,反余切arccotx,反正割arcsecx,反余割arccsc...